Section 7 – 3 Special Right Triangles Notes – Part B Properties of 30°60°90° Triangles Use the Pythagorean Theorem to complete the chart Use the right triangle below as reference A C B Write a conjecture about the relationship between the legs and hypotenuse of this type of triangle A triangle is a special right triangle (a right triangle being any triangle that contains a 90 degree angle) that always has degree angles of 30 degrees, 60 degrees, and 90 degrees Because it is a special triangle, it also has side length values which are always in a consistent relationship with one anotherSpecial Right Triangles You need to have separate sections for 45/45/90 special triangle and also, 30/60/90 special triangle Also explain if 30/60/90 and 60/30/90 triangles are same or different and why Explain how to use it to find the unknown side of another special right triangles and why you can do it Hint if two triangles are similar

Special Right Triangles Game Or Practice Problems Right Triangle Special Right Triangle Chart
Special right triangles 30 60 90 calculator
Special right triangles 30 60 90 calculator-Our new CrystalGraphics Chart and Diagram Slides for PowerPoint is a collection of over 1000 impressively designed datadriven chart and editable diagram s guaranteed to impress any audience for Right Triangles Special Cases, for Right Triangles 30 60 90 Triangles 60 30 45 45 45 90 Triangles 45 * * 2 2 2 60 60 60 30 1 1 1 An equilateralLearn how to solve for the sides in a Special Right Triangle in this free math video tutorial by Mario's Math Tutoring009 What are the Ratios of t




30 60 90 Triangle Theorem Ratio Formula Video
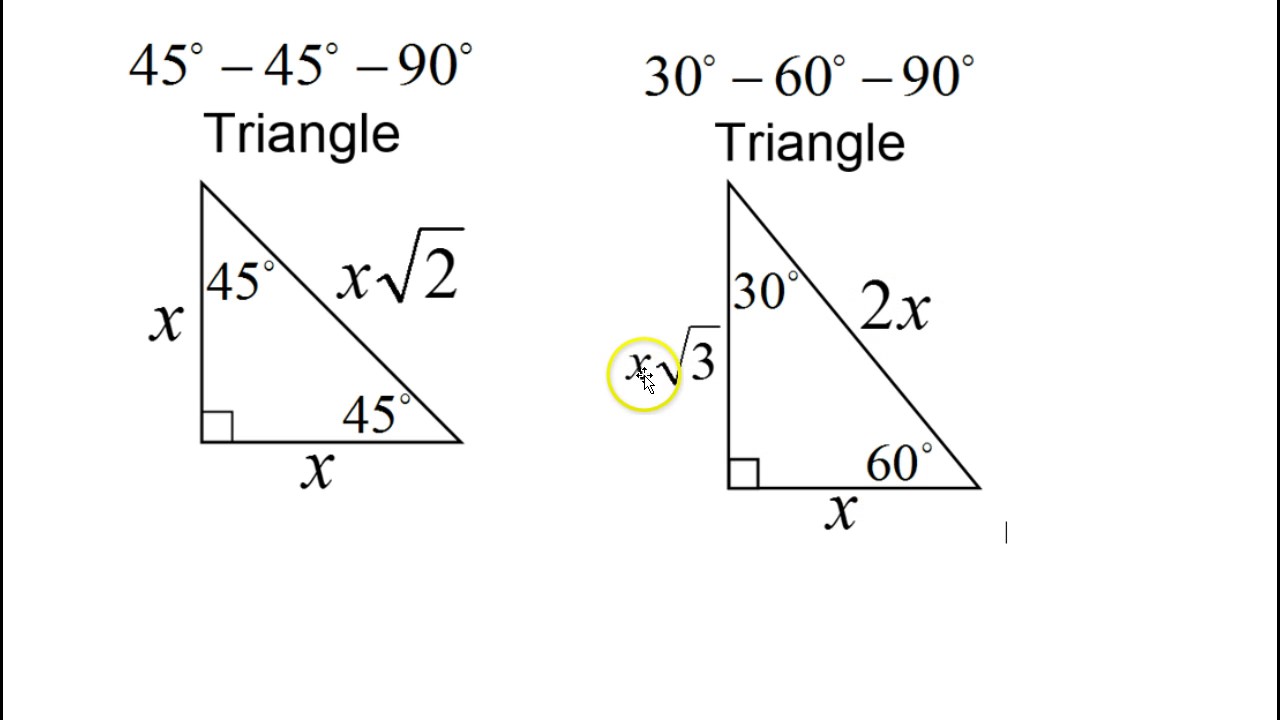
Triangle Practice Name_____ ID 1 Date_____ Period____ ©v j2o0c1x5w UKVuVt_at iSGoMfttwPaHrGex rLpLeCkQ l ^AullN Zr\iSgqhotksV vrOeXsWesrWvKe`d\1Find the missing side lengths Leave your answers as radicals in simplest form 1) 12 m n 30° 2) 72 ba 30° 3) x y 5 60° 4) x 133y 60° 5) 23 u v 60° 6) m n63 30 60 90 triangle sides If we know the shorter leg length a, we can find out that b = a√3 c = 2a If the longer leg length b is the one parameter given, then a = b√3/3 c = 2b√3/3 For hypotenuse c known, the legs formulas look as follows a = c/2 b = c√3/2 Or simply type your given values and the 30 60 90 triangle calculator will do the rest! 45° 45° 90° Triangles A right triangle with two sides of equal lengths is a 45° 45° 90° triangle The length of the sides are in the ratio of 11 √2 Leg length = 1/2 hypotenuse√2 Hypotenuse = leg√2 30° 60° 90° Triangles Hypotenuse is always opposite the right angle Short Leg is opposite the 30 angle
Special right triangles proof (part 1) CCSSMath HSGSRTC8 Google Classroom what I want to do in this video is discuss a special class of triangles called triangles and I think you know why they're called this the measures of its angles are 30 degrees 60 degrees and 90 degrees and what we're going to prove in this video thisThe following special angles chart show how to derive the trig ratios of 30°, 45° and 60° from the and special triangles Scroll down the page if you need more examples and explanations on how to derive and use the trig ratios of special angles Trigonometric Function Values Of Special Angles How to derive the trigonometric function values of 30, 45 and 60 Solving special right triangles means finding the missing lengths of the sides Instead of using the Pythagorean Theorem, we can use the special right triangle ratios to perform calculations Let's work out a couple of examples Example 1 The longer side of a 30°;
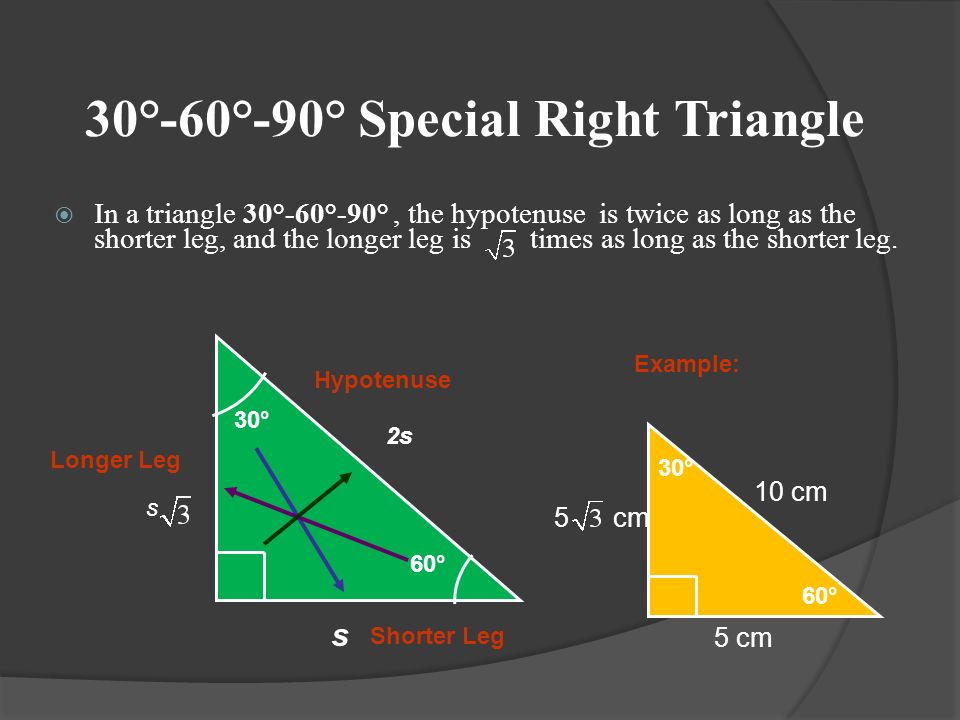
Special Triangles Isosceles and Calculator This calculator performs either of 2 items 1) If you are given a right triangle, the calculator will determine the missing 2 sides Enter the side that is known After this, press Solve Triangle 2) In addition, the calculator will allow you to same as Step 1 with a right triangleChapter 5 Completing the Triangle Toolkit 53 SPECIAL RIGHT TRIANGLES 521 522 There are two special right triangles that occur often in mathematics the 30°60°90° triangle and the 45°45°90° triangle By AA~, all 30°60°90° triangles are similar to each other, and all 45°45°90° triangles are similar to each otherTriangles Theorem 2 In a triangle whose angles measure 30 0, 60 0, and 90 , the hypotenuse has a length 0 equal to twice the length of the shorter leg, and the length of the longer leg is the product of 3 And the length of the shorter leg The ratio of the sides of a triangle are x x 3 2 x Note The short leg is always opposite the 30 ° angle!
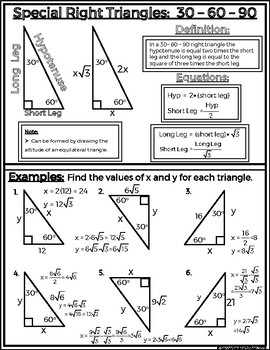



Special Right Triangles 45 45 30 60 90 Notes Sheet Graphic Organizers
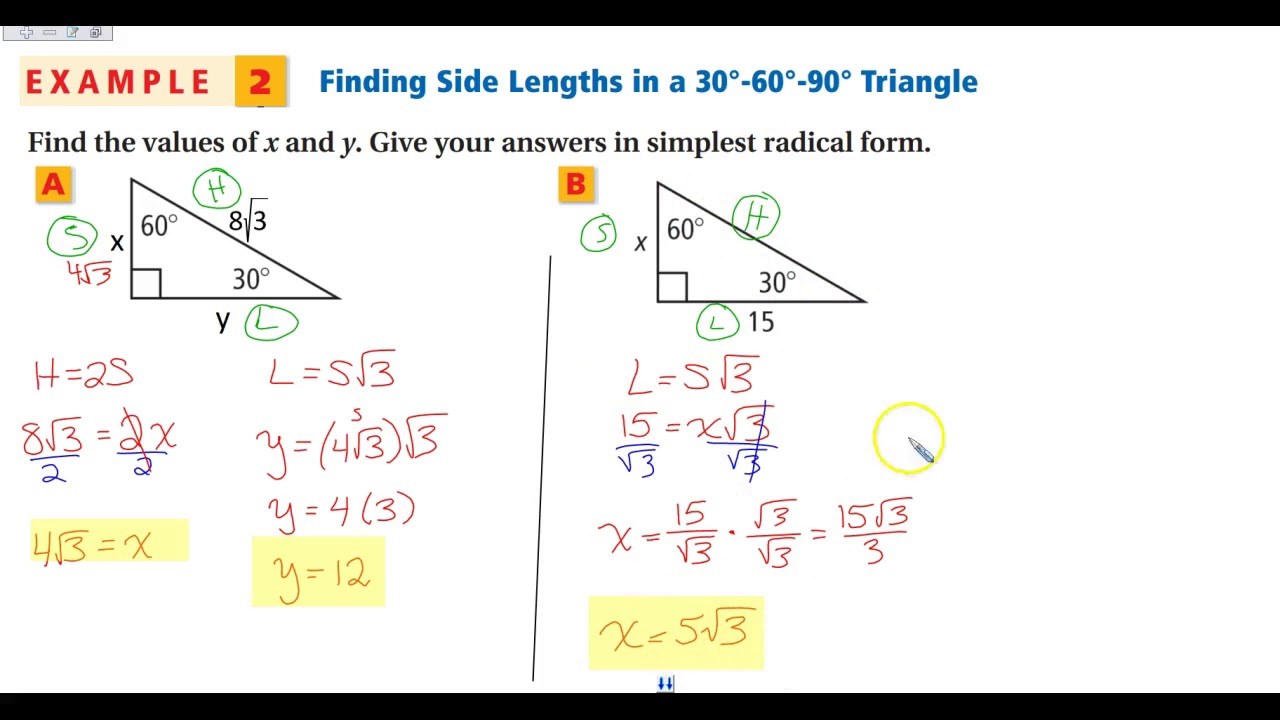



5 8b 30 60 90 Special Right Triangles Youtube
Explore Bernadette Cajigas's board "Special right triangle" on See more ideas about special right triangle, right triangle, triangle worksheetThe most frequently studied right triangles, the special right triangles, are the 30, 60, 90 Triangles followed by the 45, 45, 90 triangles The 30, 60, 90 Special Right Triangle The picture below illustrates the general formula for the 30, 60, 90 TriangleTriangles are classified as "special right triangles" They are special because of special relationships among the triangle legs that allow one to easily arrive at the length of the sides with exact answers instead of decimal approximations when using trig functions
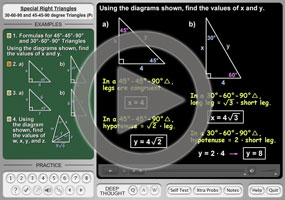



Special Angle Values 30 60 90 And 45 45 90 Triangles Purplemath




30 60 90 Triangle Theorem Ratio Formula Video
Special Right Triangles Date_____ Period____ Find the missing side lengths Leave your answers as radicals in simplest form 1) a 2 2 b 45° 2) 4 x y 45° 3) x y 3 2 2 45° 4) x y 3 2 45° 5) 6 x y 45° 6) 2 6 y x 45° 7) 16 x y 60° 8) u v 2 30°1 The shortest side of a 300 600 900 triangle is 15 Showing top 8 worksheets in the category special right triangles The hypotenuse of a 300 600 900 triangle is 18 One leg of a 450 450 900 triangle is 9 The most frequently studied right triangles the special right triangles are the 30 60 90 triangles followed by the 45 45 90 trianglesPreCalculus Course https//wwwyoutubecom/c/MrHelpfulNotHurtful/playlists?view=50&sort=dd&shelf_id=3How do we find the unknown sides of special right tria




Day 1 Hw Special Right Triangles 45 45 90 30 60 90 Youtube Right Triangle Trigonometry Worksheets Triangle Worksheet




Special Right Triangles Game Or Practice Problems Right Triangle Special Right Triangle Chart
Multiply this answer by the square root of 3 to find the long leg Type 3 You know the long leg (the side across from the 60degree angle) Divide this side by the square root of 3 to find the short side Double that figure to find the hypotenuse Finding the other sides of a triangle when you know the hypotenuse A 30 60 90 triangle is a special type of right triangle 30 60 90 triangle practice What is special about 30 60 90 triangles is that the sides of the 30 60 90 triangle always have the same ratio Special right triangles use the 30 60 90 and 45 45 90 triangle relationships to solve for the missing sidesSpecial Right Triangles A special right triangle is one which has sides or angles for which simple formulas exist making calculations easy Of all these special right triangles, the two encountered most often are the 30 60 90 and the 45 45 90 triangles For example, a speed square used by carpenters is a 45 45 90 triangle




Mrs Newell S Math Better Questions Special Right Triangles



30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com
Special Right Triangles 30°60°90° triangle The 30°60°90° refers to the angle measurements in degrees of this type of special right triangle In this type of right triangle, the sides corresponding to the angles 30°60°90° follow a ratio of 1√ 32 Thus, in this type of triangle, if the length of one side and the side'sAnother type of special right triangles is the 30°60°90° triangle This is right triangle whose angles are 30°60°90° The lengths of the sides of a 30°60°90° triangle are in the ratio of 1 √3 2 You can also recognize a 30°60°90° triangle by the angles As long as you know that one of the angles in the rightangle triangle is either 30° or 60° then it must be a 30°60°90°A = 245 mm 30°60°90° Triangles Consider an equilateral ∆ 2x x 2x x a 60° 60° 30° a2 = (2x)2 – x2 Simplify a2 = 4x2 – x2 a2 = 3x2 √a2 = √3x2 a = x√3



Objectives Use Properties Of 45 45 90 Triangles Use Properties Of 30 60 90 Triangles Ppt Download




Special Right Triangle 30 60 90 Mathbitsnotebook Geo Ccss Math
A triangle is a right triangle with angle measures of 30º, 60º, and 90º (the right angle) Because the angles are always in that ratio, the sides are also always in the same ratio to each other The side opposite the 30º angle is the shortest and the length of itThese activity cards include special right triangles There are 18 cards for and 9 cards that cover They can be printed on cardstock for durability Place page 1 and 3, then 2 and 4 together and print 2 sided for correct flashcards Perfect I can use the properties of and triangles to find missing side lengths title "Special Right Triangles (, )" 19 by user Linda Gregory under license "Creative Commons Attribution NonCommercial" Version History Cite this work




Special Right Triangles Review Article Khan Academy
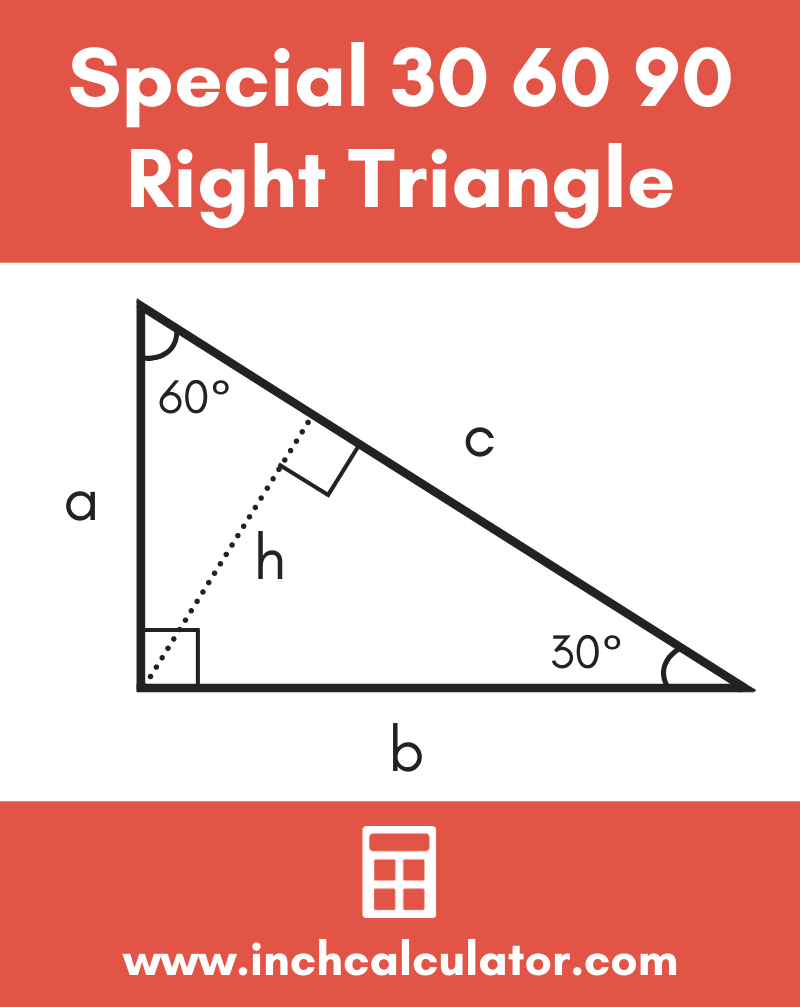



30 60 90 Special Right Triangle Calculator Inch Calculator
The and Triangles It is wellknown that an altitude splits an equilateral triangle into two triangles, and that a diagonal splits a square into two triangles The properties of these "special right triangles," as they are often called, are wellunderstood, and shall not be described here90° right triangle is given by 8√3 cmIsosceles Right Triangles 14 30°60°90° 15 Mixed practice 1617 Trigonometry 18 Trigonometry 21 Holiday 22 Trigonometry 2324 REVIEW Begin Test 25 TEST Tuesday, 1/8 Pythagorean Theorem 1 I can solve for the missing hypotenuse of a right triangle 2 I can solve for the missing leg of a right triangle 3 I can identify Pythagorean Triples
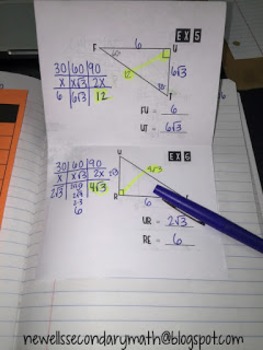



Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math




Special Right Triangles Video Lessons Examples And Solutions
The most frequently studied right triangles the special right triangles are the 30 60 90 triangles followed by the 45 45 90 triangles 14 the length of one side of an equilateral triangle is 6meters Find the lengths of the other sidesOnce the students are comfortable with the , we will watch a video explaining the triangle I will demonstrate a few on the board and the students will then independently complete 6 of the problems on the sheet called Special Right TrianglesUse the Pythagorean theorem to discover patterns in 30°60°90° and 45°45°90° triangles




Mrs Newell S Math Better Questions Special Right Triangles
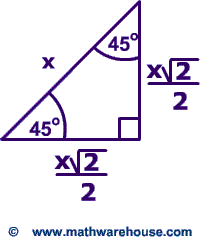



Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems
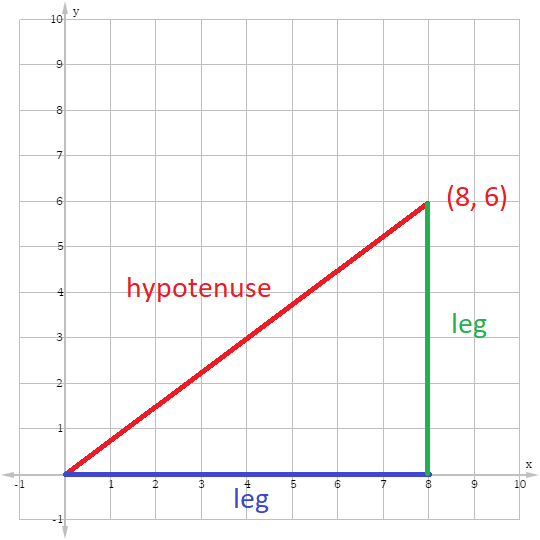
Similar Triangles Special right triangles and within triangle ratios triangles Triangle ABC below is equilateral The altitude from vertex B to the opposite side divides the triangle into two right triangles (a) Is ABC ≅ CBD?A triangle is a right triangle where the three interior angles measure 30 °, 60 °, and 90 ° Right triangles with interior angles are known as special right triangles Special triangles in geometry because of the powerful relationships that If this triangle is a special right triangle, then we can solve for the side lengths and figure out the coordinates!
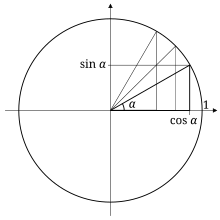



Special Right Triangle Wikipedia
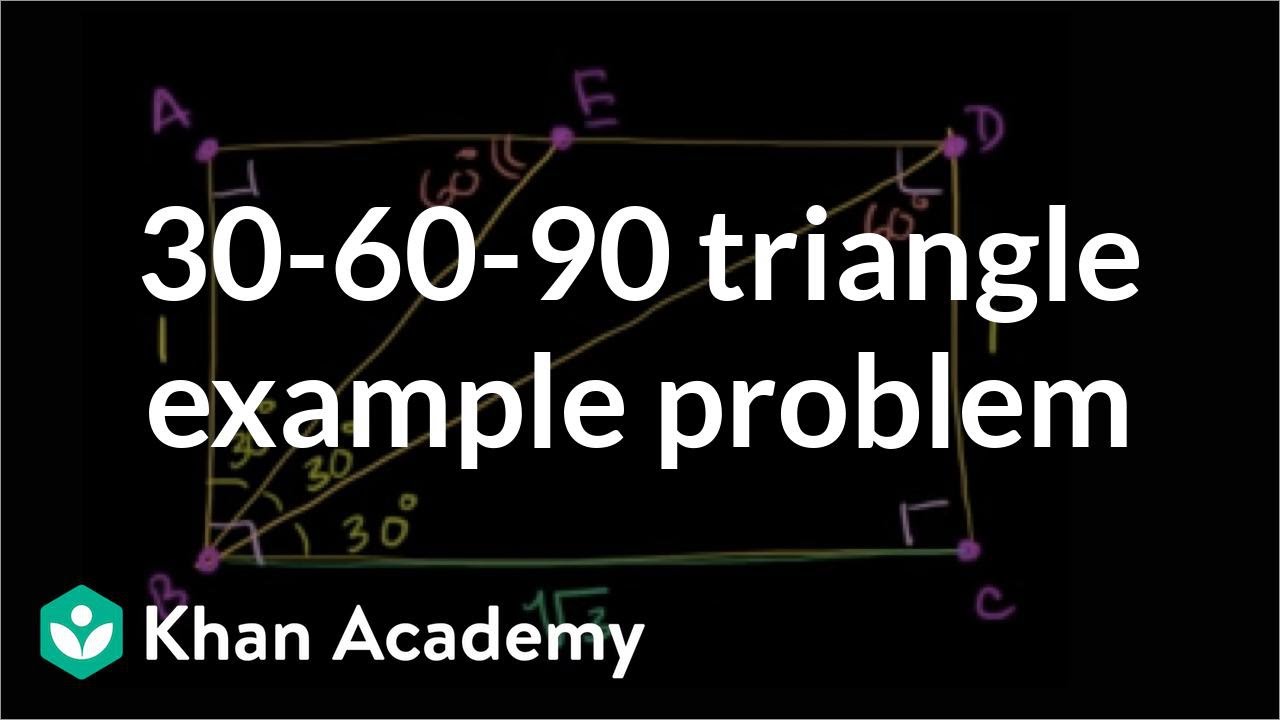



30 60 90 Triangle Example Problem Video Khan Academy
Special right triangles Chart Booklet Theorem Theorem MGSE912GSRT6 Understand that by similarity, side ratios in right triangles are properties of the angles in the triangle, leading to definitions of trigonometric ratios for acute angles MGSE912GSRT8 Use trigonometric ratios and the Pythagorean Theorem to solve right triangles in appliedA right triangle (literally pronounced "thirty sixty ninety") is a special type of right triangle where the three angles measure 30 degrees, 60 degrees, and 90 degrees The triangle is significant because the sides exist in an easytoremember ratio 1 √3 3 2 That is to say, the hypotenuse is twice as long as the shorter leg, andA special right triangle is a right triangle with some regular feature that makes calculations on the triangle easier, or for which simple formulas exist For example, a right triangle may have angles that form simple relationships, such as 45°–45°–90° This is called an "anglebased" right triangle A "sidebased" right triangle is one in which the lengths of the sides form ratios of




Mrs Newell S Math Better Questions Special Right Triangles




30 60 90 Right Triangle Side Ratios Expii
Explain (b) What are the lengths of AD and DC?Example Problems Problem 1 First, let's use the right triangle to unify our subjects Using a right triangle, calculate the cosecant of 45 degrees without referring to the chart above Problem 1 Show AnswerSimilar Triangles Special right triangles and within triangle ratios triangles Triangle ABC below is equilateral The altitude from vertex B to the opposite side divides the triangle into two right triangles (a) Is ABC ≅ CBD?
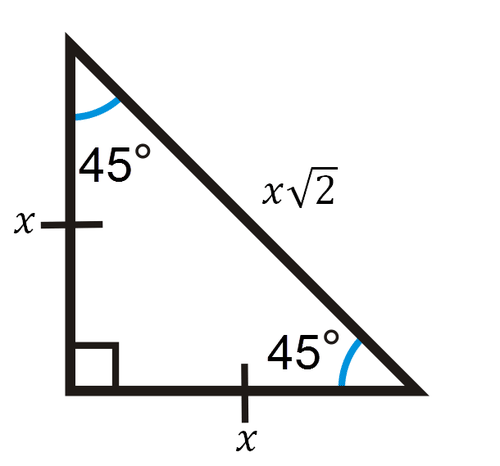



Special Right Triangles Ck 12 Foundation
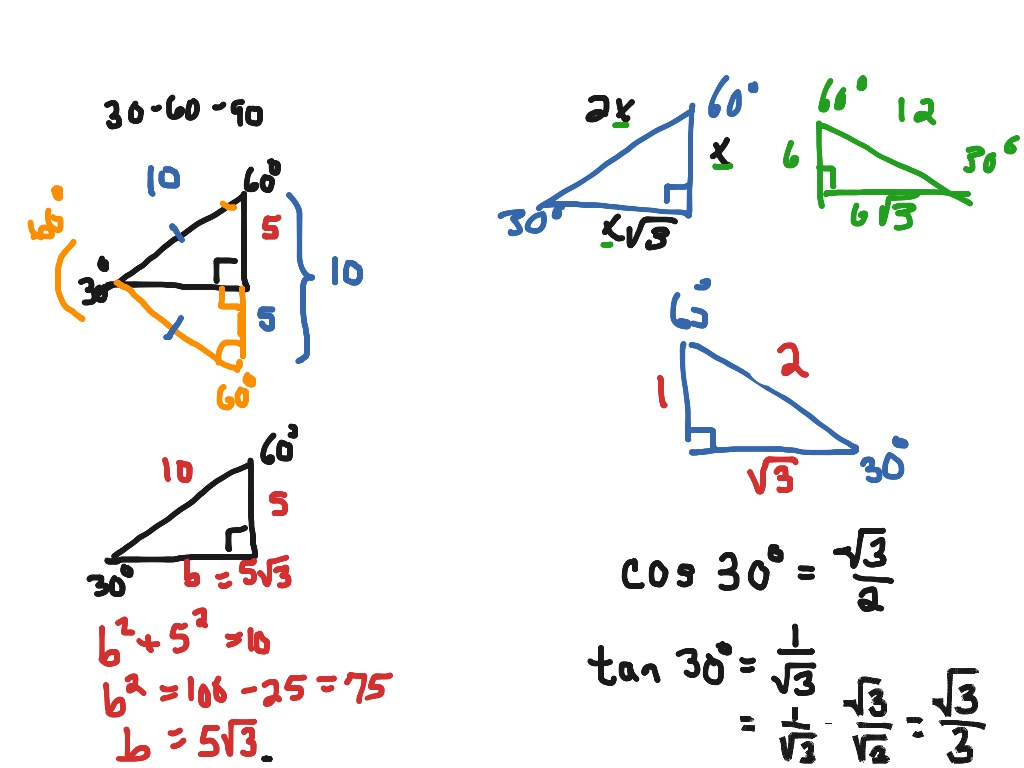



30 60 90 Right Triangles Math Trigonometry Trig Triangles Right Triangles Showme
Finding Exact Values of Trig Ratios Special Right Triangles Special Right Triangles Consider the Special Angles in relation to the Unit Circle – A free PowerPoint PPT presentation (displayed as a Flash slide show) on PowerShowcom id 78f552NWQ0M




30 60 90 Triangle Theorem Ratio Formula Video
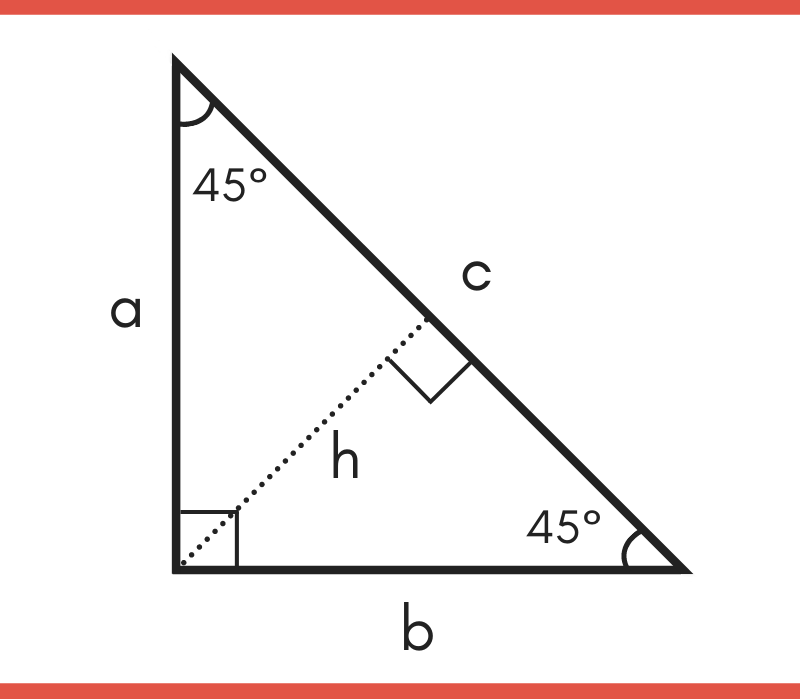



45 45 90 Special Right Triangle Calculator Inch Calculator



1




30 60 90 Right Triangles Solutions Examples Videos




30 60 90 Triangle Theorem Ratio Formula Video
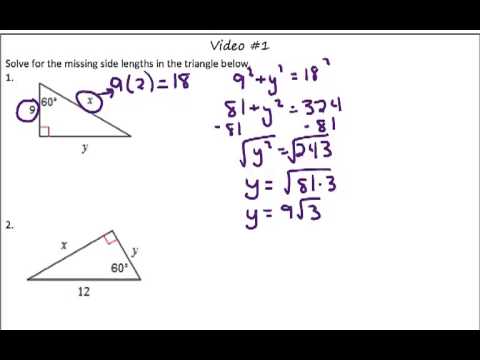



Special Right Triangles 30 60 90 Video 1 Youtube




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation
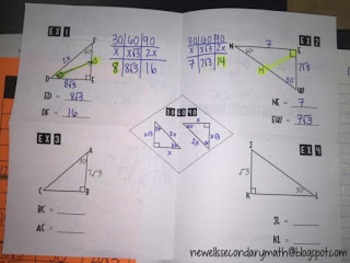



Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math



30 60 90 Triangle Formulas Rules And Sides Science Trends



30 60 90 And 45 45 90 Triangle Calculator




I Teach Special Right Triangles Using Proportions My Students Create 2 Notecards One With A 45 45 90 Unit Tria Special Right Triangle Math Methods Math Words



1



Day 16 Test C 9 To 12 Special Right Triangles 30 60 90 45 45 90 Youtube



30 60 90 And 45 45 90 Triangle Calculator




Special Right Triangles Anchor Chart Math Interactive Notebook Fractions Anchor Chart Math Interactive
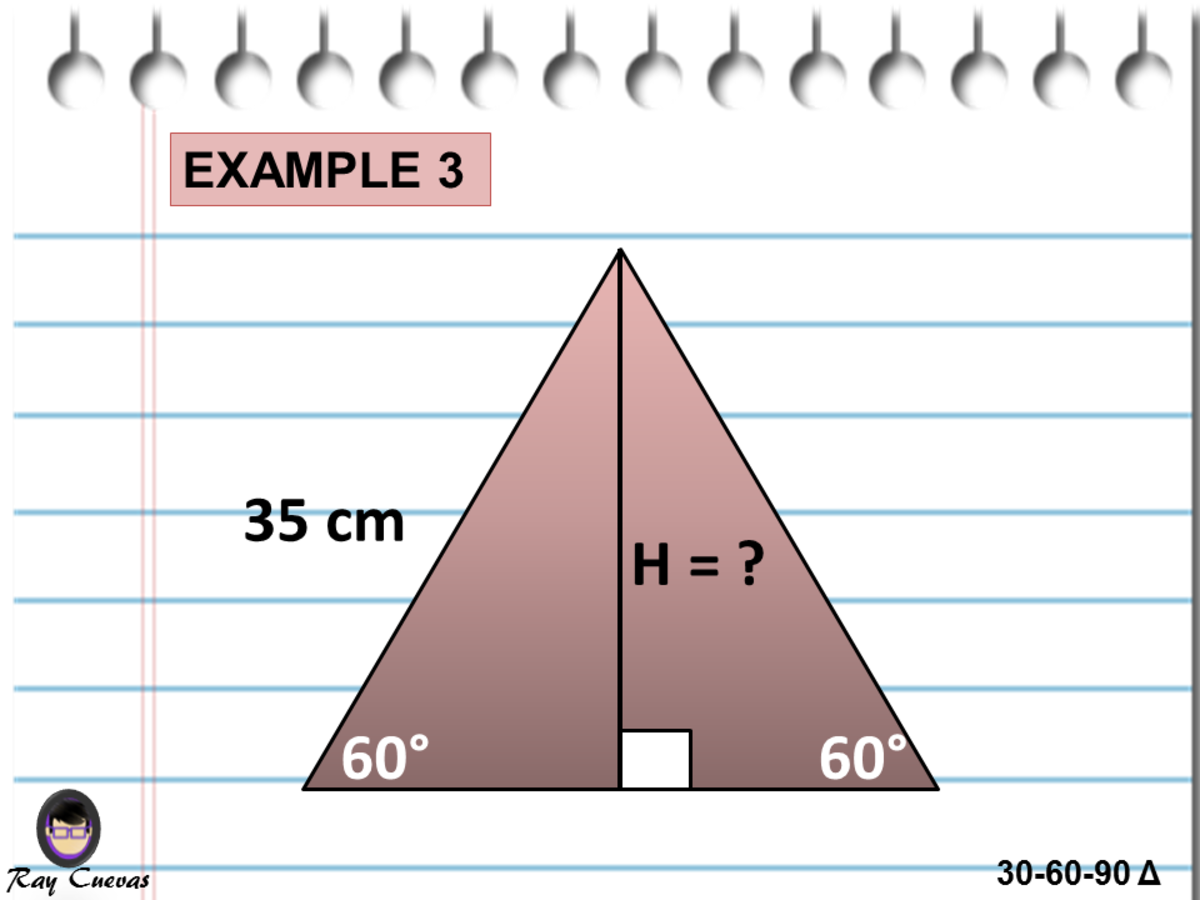



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Right Triangles Free Math Help
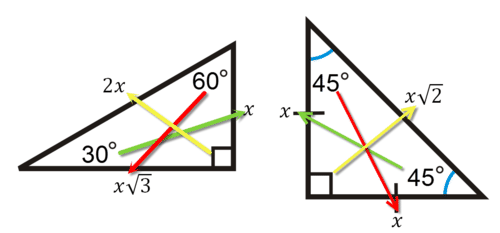



Special Right Triangles Ck 12 Foundation
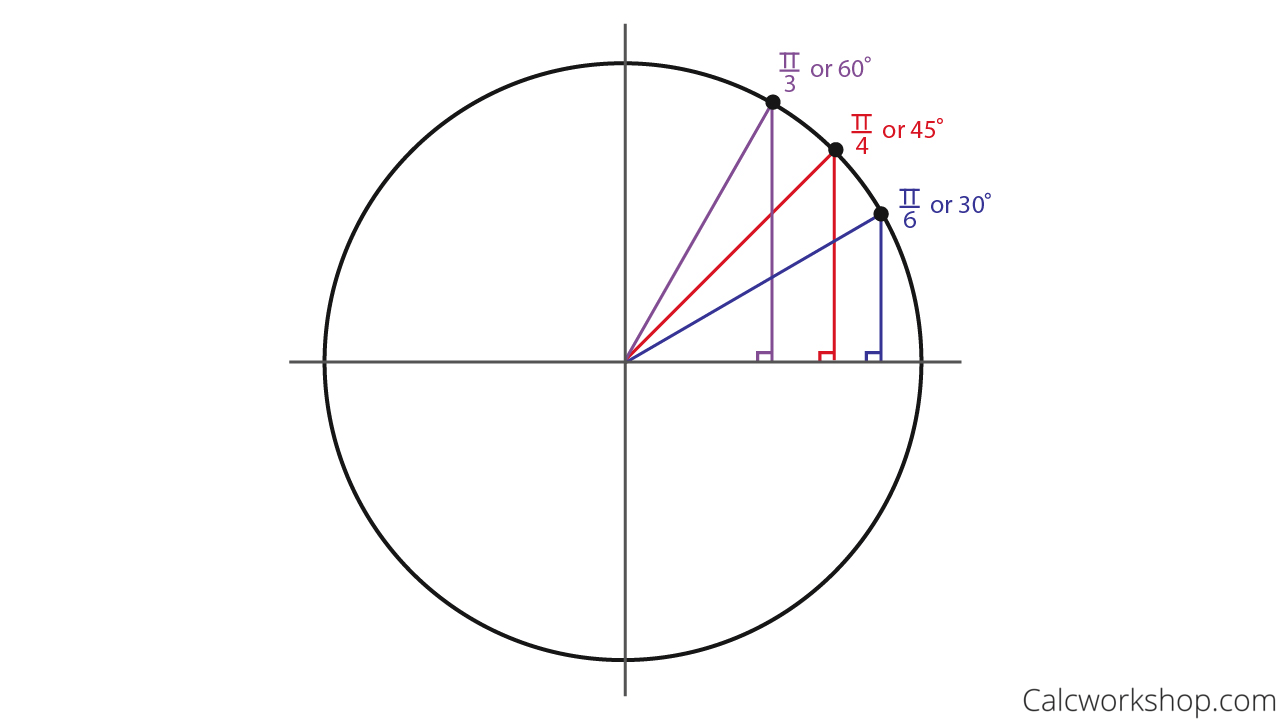



Unit Circle W Everything Charts Worksheets 35 Examples
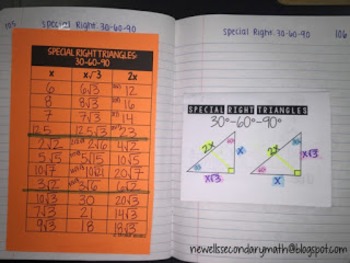



Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math




30 60 90 Right Triangles Ck 12 Foundation




30 60 90 Special Right Triangle Diagram Quizlet
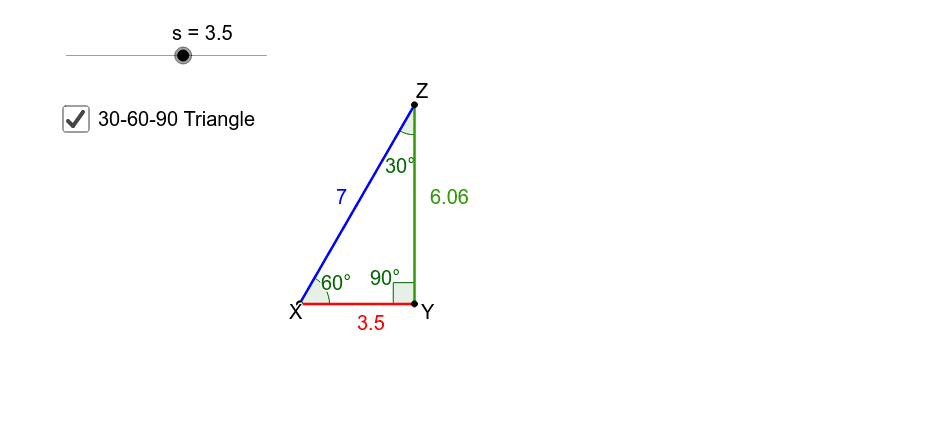



Special Right Triangles 30 60 90 Geogebra




30 60 90 Special Right Triangle Calculator Inch Calculator




30 60 90 Triangle Explanation Examples
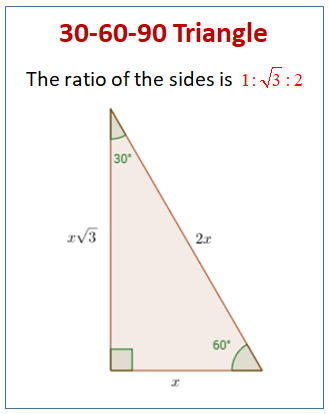



30 60 90 Special Right Triangles Videos Worksheets Examples Solutions




How To Work With 30 60 90 Degree Triangles Education Is Around
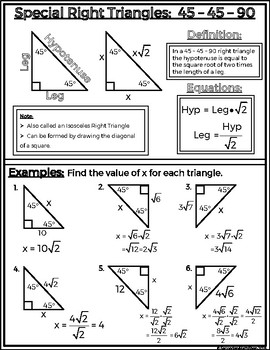



Special Right Triangles 45 45 30 60 90 Notes Sheet Graphic Organizers



Discovering Special Right Triangles 30 60 90 Triangles Made With Medians Of Equilateral Triangles Activity Builder By Desmos



Special Right Triangles Teacher Guide




How Do You Find Trigonometric Ratios Of 30 45 And 60 Degrees Socratic
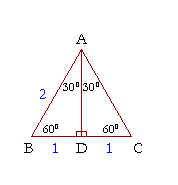



30 60 90 Right Triangle Side Ratios Expii




30 60 90 Triangle Calculator Formula Rules
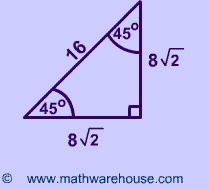



Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems




Trig Values For Paper 1 Triangle Method Gcse
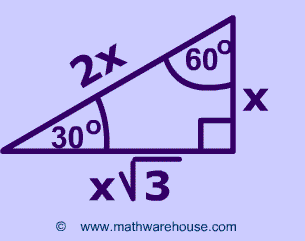



Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems
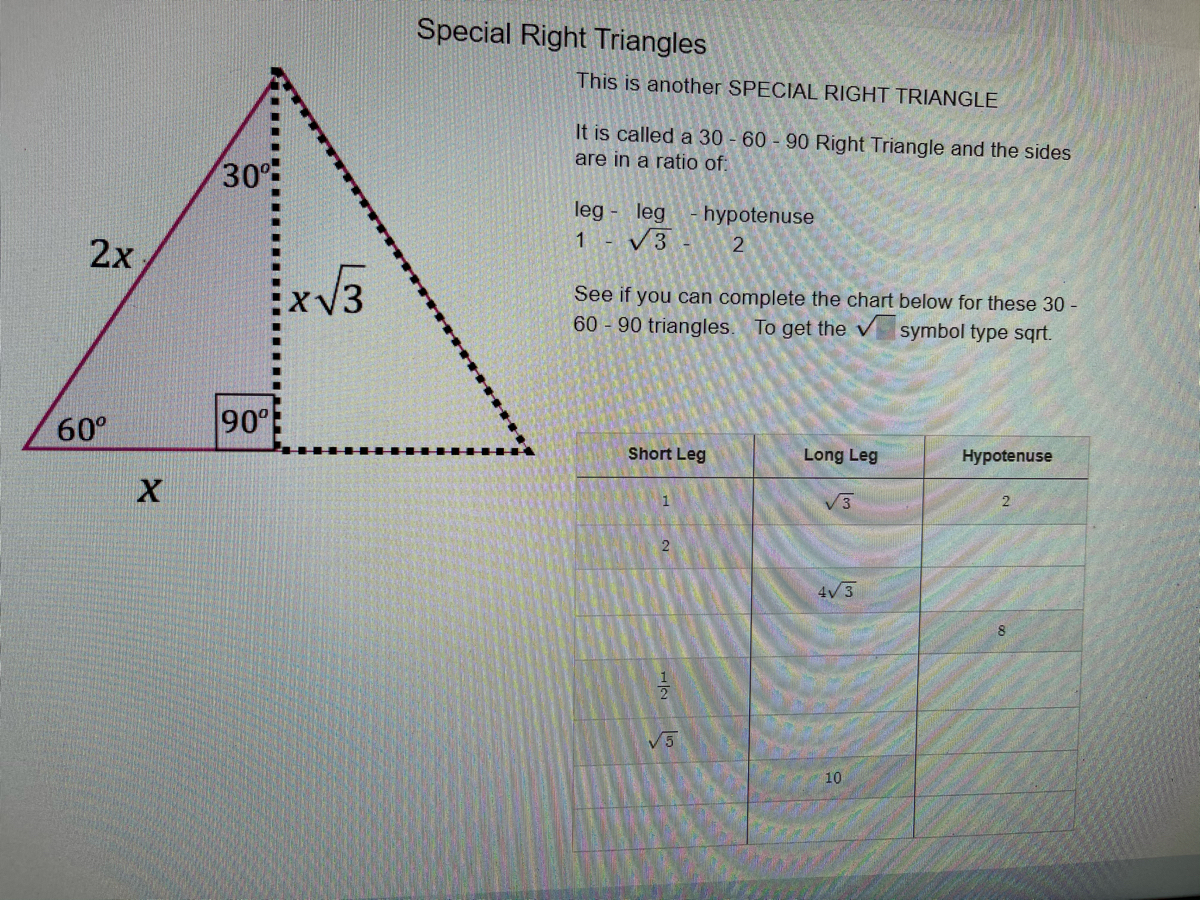



Answered Special Right Triangles This Is Another Bartleby




How To Work With 30 60 90 And 45 45 90 Degree Triangles Dummies




30 60 90 Triangle Theorem Ratio Formula Video



The Easy Guide To The 30 60 90 Triangle



1



30 60 90 And 45 45 90 Triangle Calculator




30 60 90 Triangle Explanation Examples




The Complete Guide To The 30 60 90 Triangle




30 60 90 Triangle Theorem Ratio Formula Video




Special Right Triangle Wikipedia



Biomath Trigonometric Functions



Special Right Triangles



1




30 60 90 Triangle Formulas Rules And Sides Science Trends
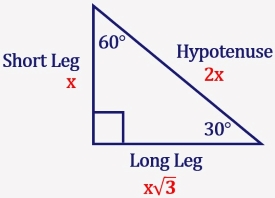



Special Right Triangle 30 60 90 Mathondemand Com



30 60 90 Triangles Systry



Special Right Triangles



Biomath Trigonometric Functions
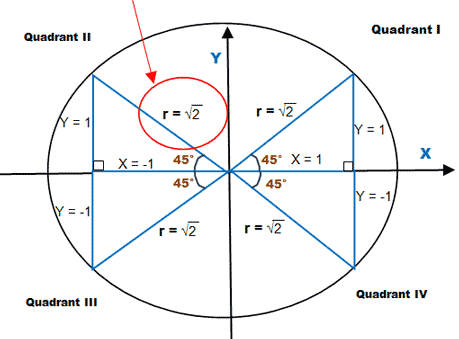



Trigonometry Snow Mountain
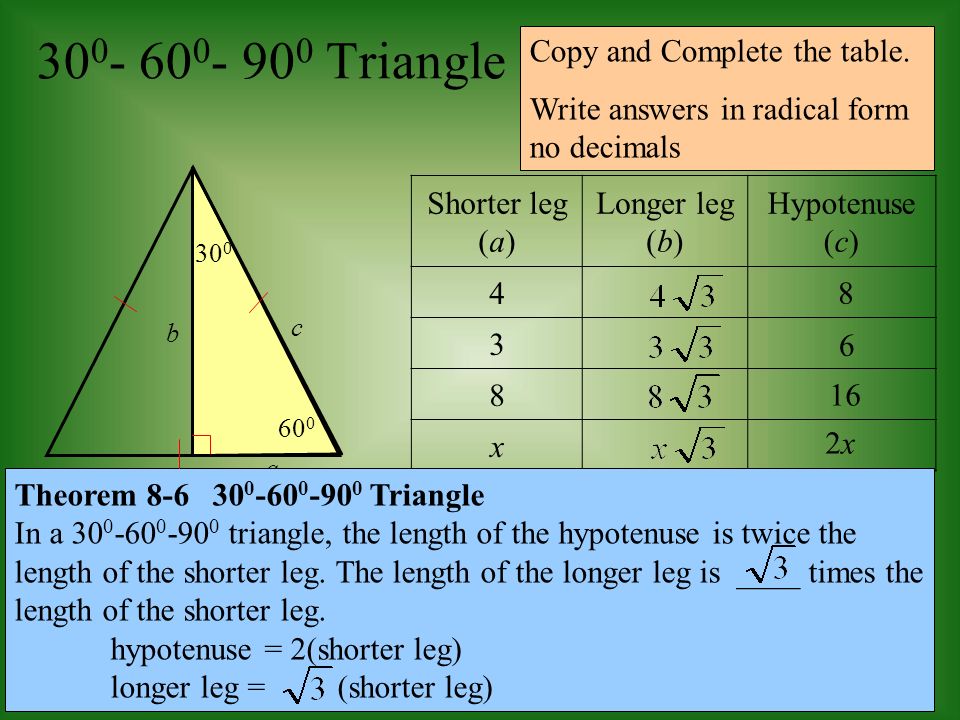



8 2 Special Right Triangles Objective To Use The Properties Of And Triangles Ppt Download




Unit Circle Chart
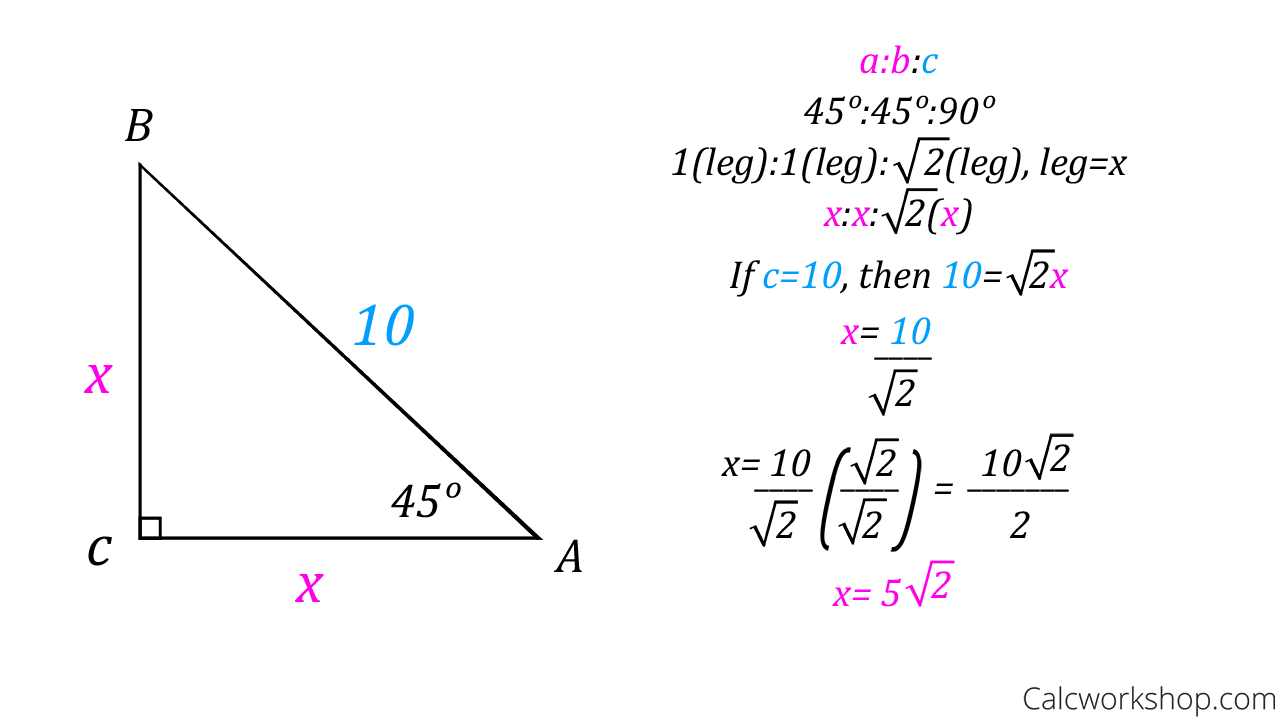



Special Right Triangles Fully Explained W 19 Examples
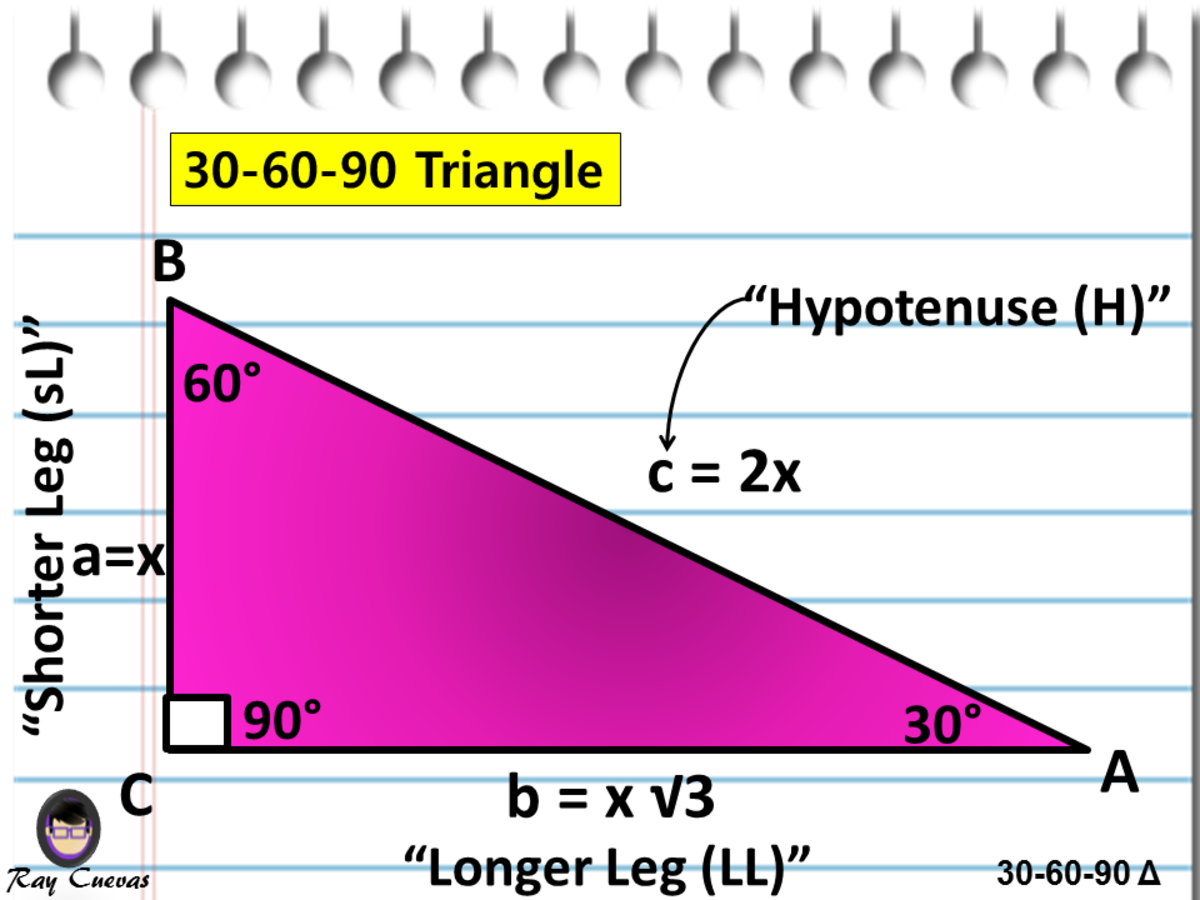



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




Mrs Newell S Math Better Questions Special Right Triangles



Special Right Triangles Activity Builder By Desmos



45 45 90 And 30 60 90 Triangles Zona Land Education
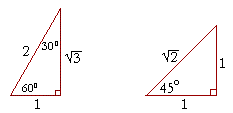



The 30 60 90 Triangle Topics In Trigonometry




The Complete Guide To The 30 60 90 Triangle
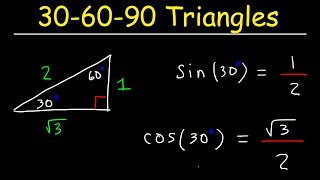



30 60 90 Triangles Special Right Triangle Trigonometry Youtube




Special Right Triangles Middle School Geometry Anchor Charts Are In The Work Middle School Geometry Geometry Anchor Chart Middle School Anchor Charts



Special Triangles Unit Circle S Tlcox S Portfolio
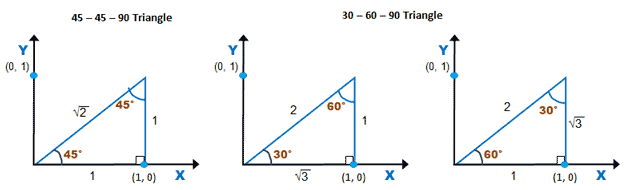



Trigonometry Snow Mountain



The Easy Guide To The 30 60 90 Triangle
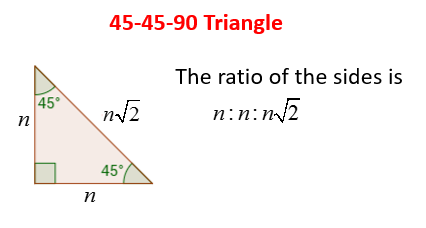



45 45 90 Right Triangles Solutions Examples Videos




30 60 90 Triangle Calculator Formula Rules




30 60 90 Right Triangles Ck 12 Foundation
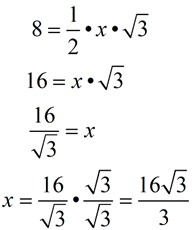



Special Right Triangle 30 60 90 Mathbitsnotebook Geo Ccss Math




A Quick Guide To The 30 60 90 Degree Triangle Dummies




Special Right Triangles 30 60 90 And 45 45 90 Triangles Youtube




Properties Of 3 4 5 Triangles Definition And Uses Video Lesson Transcript Study Com




30 60 90 Triangle Explanation Examples



The Easy Guide To The 30 60 90 Triangle
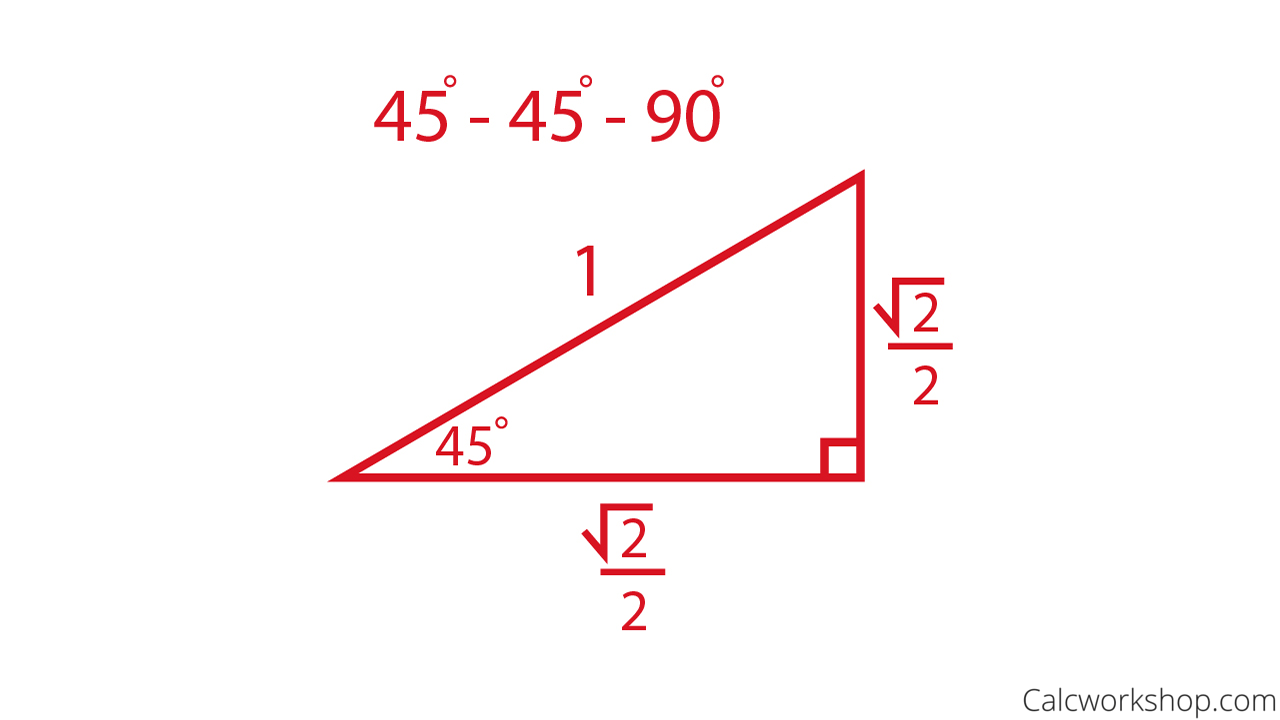



Unit Circle W Everything Charts Worksheets 35 Examples
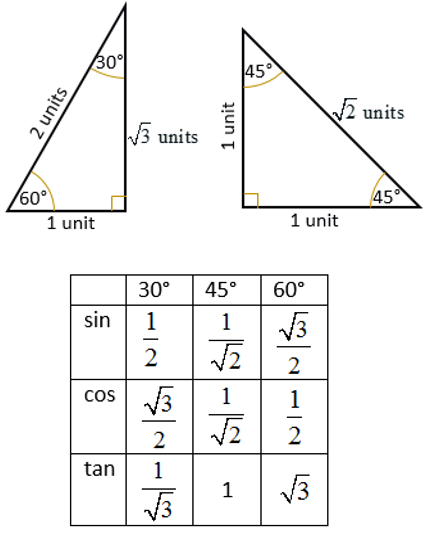



Trigonometric Ratios Of Special Angles 0 30 45 60 90 Video Lessons Examples And Solutions




Special Right Triangles Fully Explained W 19 Examples




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation



0 件のコメント:
コメントを投稿